Assignment on Earthquake Super Cycle

Guess the Length of the super cycle (time interval between the two successive M-9 earthquakes) assuming that the earthquake fully releases the stress associated with the Pacific plate subduction.

Ans:

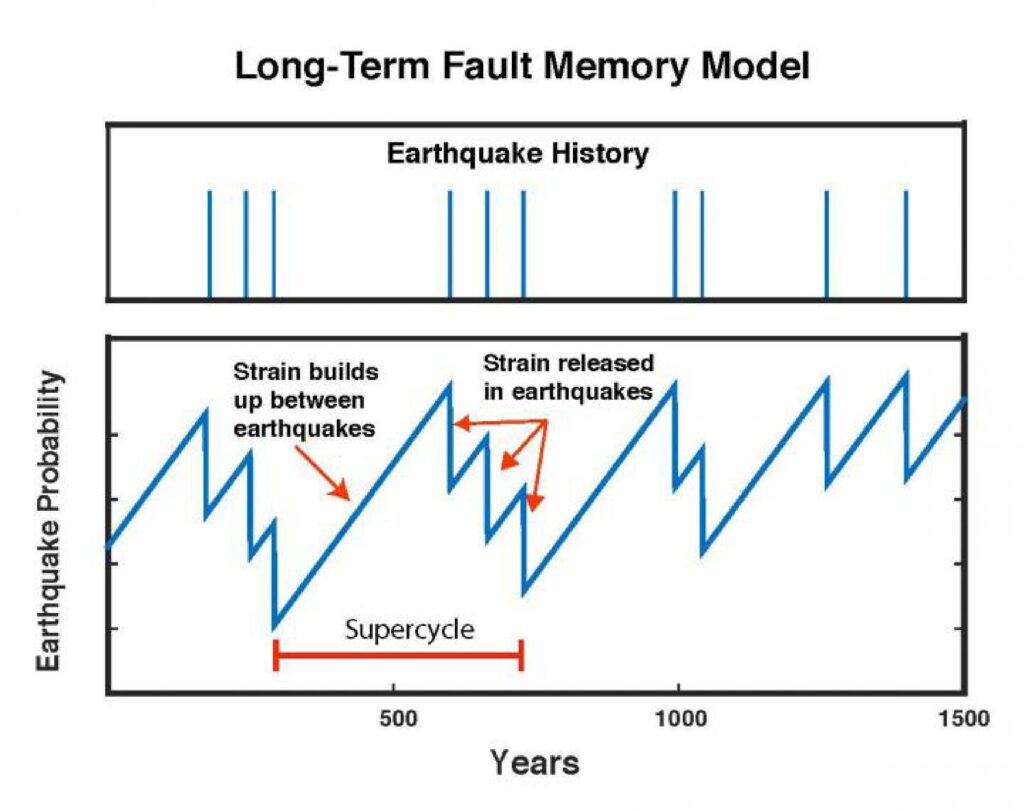
From the New model explaining the coexistence of frequent M-8 and infrequent M9 earthquakes we find that, to complete one Super cycle requires approximately 571 years because, from the past record, we know that, in 869 BC, 1611 BC? and in 2011 BC there are three successive large earthquakes occurred and completing three supercycles by releasing all strain of strong coupling.

According to this new model, when the Pacific plate subducts beneath the North American continental plate all strain is not released by small asperities but accumulated for a long time. Then by releasing the entire strain a large infrequent earthquake (M9) is occurred and completes a super cycle.

So, from the new model;

{(1611-869)+(2011-1611)}/2

=571 year

From the above conclusion we can guess the length of the super cycle is 571 years.

Assignment on Earthquake Super Cycle: Q2

Guess the amount of coseismic slip associated with the usual M-8 earthquake, assuming that the seismic coupling coefficient is 0.8 and the recurrence time interval is 100 year

Ans;

We know, the coupling  coefficient, x=D/vT₀ 

>  80%=D/8*100

Therefore D=640 cm=64 meter (ans).

Where,

X= coupling  coefficient= 0.8=80%

D=coseismic slip

V=motion of plate =8 cm/year

T₀=Recurrence interval=100 years

So, we can guess the amount of coseismic slip (D) is 64 m.