What is the Gravity Anomaly Equation

Gravity Anomaly: A difference between the locally observed and the theoretically calculated value of gravity that reflects local variations in the density of underlying rocks and is often helpful in geophysical prospecting.

Gravity anomalies are the difference between the observed acceleration of the earths gravity and the values predicted from some model of how the gravity would be predicted to appear.　The gravity anomalies at a location on the earths surface is the difference between the observed value of gravity and the value predicted by a theoretical model.　

The three most commonly used gravity anomalies are:

1.Free-air or faye gravity anomaly

2.the Bouger gravity　anomaly

3. the isostatic gravity anomaly.

Other varieties of gravity anomalies have been　propoesd,　for example the Rudzki inversion and helmert condensation gravity anomalies but these are seldom used.

1. Free- air anomaly:

In geophysics free- air gravity anomaly often called the free-air anomaly.Free –air anomaly is the measured gravity anomaly after a free-air correction is applied to account for the elevation at which a measurement is made.

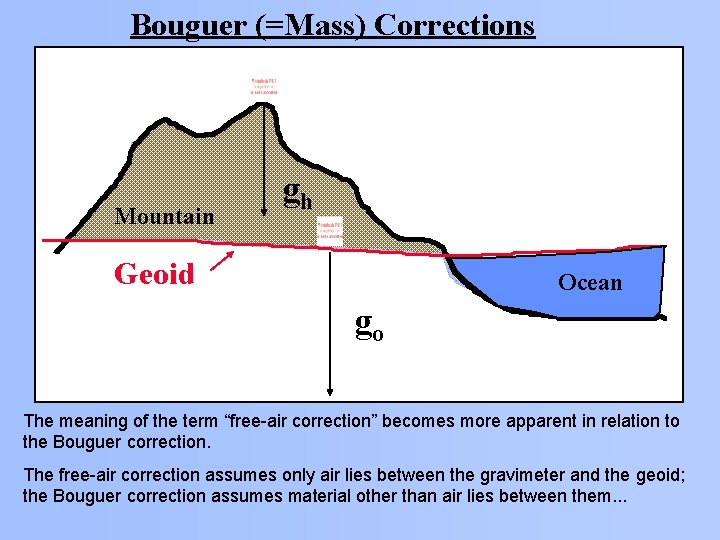
2. Bouguer anomaly:

The Bouguer anomaly is the difference between the expected value of gravity at a given location(taking into account factors such as latitude,　longitude,　altitude and the rotation of the earth) and its actual value.　Bouguer anomalies can be measured in several ways depending whether the density and shape of the
terrain between the measuring point and sea level is calculated,　estimated or ignored.

3. Isostatic gravity anomaly:

Isostatic gravity anomalies are alleged to provide a measure of the earths gravity field free from the gravitational attractions of the topography and its isostatic
compensation. Most commonly represented by a variation in the depth of a compensating density contrast for example the moho.

Gravity anomalies are often due to unusual　concentrations of mass in a region. For example,　the presence of mountain ranges will usually cause the gravitational force to be more than it would be on a featureless planet.-positive anomaly. A positive anomaly is reading that exceeds the average magnetic field strength and is usually related to more strongly magnetic rocks,such as mafic rocks or magnetic-bearing rocks, underneath the magnetometer.

A negative magnetic anomaly is areading that is lower than the average magnetic field. Negative anomalies are interested to mean that the segments of the lithosphere( that is the crust and upper mantle comprising the rigid, outermost shell of the earth) that underlie trenches are being forced down against buoyant isostatic forces.

In geodesy and geophysics, the bouguer anomaly (named after pierre Bouguer) is a anomaly, corrected for a hight at which it is measured and the attraction of terrain.The heighet
correction alone gives a free-air gravity anomaly. Otherwise the bouguer reduction does not work properly.

The equation used to calculate a gravity anomaly depends on the specific situation and the simplifying assumptions made. Here’s a breakdown of two common scenarios:

1. Gravity Anomaly due to a Buried Mass:

This scenario considers a point mass (a small, spherical object) buried within a uniform Earth. The vertical component (vertical direction) of the gravity anomaly caused by this mass, denoted by gz, can be calculated using the following equation:

gz = G * ∫ (dm / r²) * cos(θ)

where:

• G is the gravitational constant (approximately 6.6743 × 10^-11 m³ kg⁻¹ s⁻²)
• dm is a tiny element of mass within the buried object
• r is the distance between the element of mass (dm) and the point where gravity is measured
• θ is the angle between the center of the mass, the element of mass (dm), and the point where gravity is measured (usually defined relative to the vertical direction)
• ∫ represents the integral over the entire mass of the object

This equation essentially calculates the gravitational attraction of each tiny piece of mass (dm) within the buried object, considering the distance (r) and the angle (θ), and then sums them all up using the integral.

Simplifying Assumptions:

For most practical applications, simplifying assumptions are made to obtain a more manageable equation. Here’s a common simplification:

• The buried object is assumed to be a sphere with a uniform density.
• This allows us to replace the integral with a single term that considers the object’s total mass (M), its radius (a), and the depth (z) from the surface to the center of the sphere.

With these simplifications, the equation for the vertical component of the gravity anomaly due to a buried sphere becomes:

gz = (4 * π * G * Δρ * a^3) / (3 * (x^2 + z^2)^(3/2))

where:

• Δρ (Delta Rho) is the density contrast between the buried object and the surrounding material (kg m^-3)
• a is the radius of the sphere (m)
• x is the horizontal distance from the center of the sphere to the point where gravity is measured (m)
• z is the depth from the surface to the center of the sphere (m)

2. Bouguer Anomaly:

The Bouguer anomaly is a commonly used gravity anomaly in geophysical exploration. It considers the excess mass causing the anomaly relative to a reference ellipsoid (a simplified Earth model). The equation for the Bouguer anomaly (Δgb) depends on the specific assumptions and corrections applied. Here’s a simplified version:

Δgb = - G * ρ * h

where:

• Δgb is the Bouguer anomaly (mgal)
• G is the gravitational constant
• ρ is the density of the crustal rock (usually assumed to be around 2670 kg m^-3)
• h is the elevation above the reference ellipsoid (m)

Important Note:

These are simplified representations, and the actual equations used in practice can be more complex depending on the specific situation and the desired level of accuracy. It’s important to consult geophysical references and software for the most appropriate equation and data analysis techniques for your specific application.